Mechanism of Enhanced Persistence in the Source-Sink Coupled Map System

So what is the underlying mechanism ? Is it "spreading of risk" ?

If that was so, even coupling two source habitat maps (i.e., both having a = 4) would have given us enhanced persistence ?
But that is not true.

Coupling two source habitat maps together with strong coupling synchronizes them rapidly and the combined system effectively reduces to a single source habitat map - with a very low persistence time.

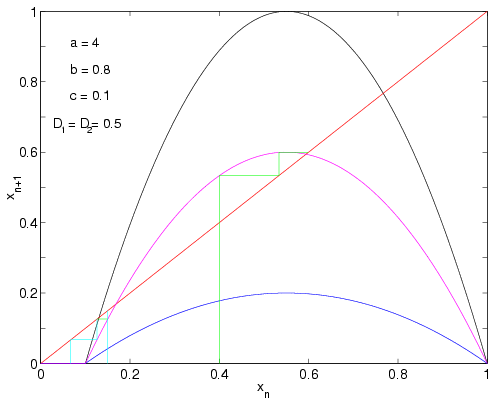
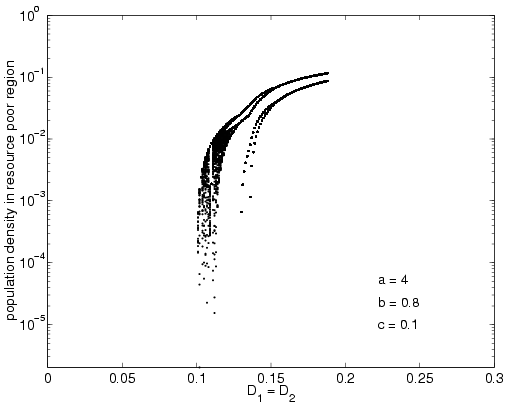
The strong coupling limit (D = 0.5)
If migration (i.e., risk spreading) was the only factor enhancing persistence we should see persistence at this limit for all values of c.

At this limit, it is very easy to see the effect of the coupling - the resultant map of the average population of the two habitats is simply the average of the two individual maps.

Two features to note:

At intermediate values of c, multiple attractors coexist with one of the attractors being x = 0.
At high values of c, the only attractor is at x = 0. So for high values of c, even the strongest coupling cannot produce enhanced persistence.

Multiple coexistent attractors - initial value dependence of persistence

History does matter: The initial value of the populations specify whether the coupled system will be eventually persistent.

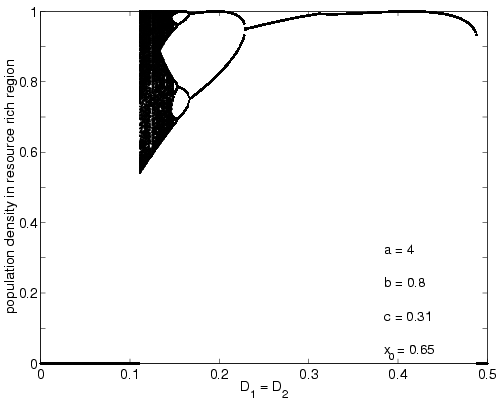
The mechanism: Nonlinearity

The effective map due to the coupling is altered from the unperturbed map - such that it no longer has any region mapping to the trapping region.

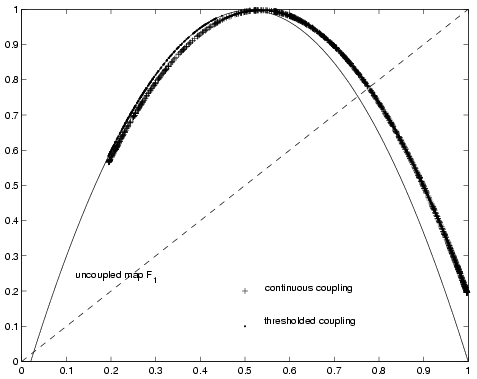
The persistence time is infinite.

So far we had been looking at the system under the (simple but ecologically unrealistic) conditions of:

symmetric migration, and,
the absence of habitat selection

Let us look at the case of asymmetric migration.
It turns out that the value of D₂ is not critical in determining the persistence of the coupled system (other parameters being kept fixed).
So, only the source to sink migration rate matters!

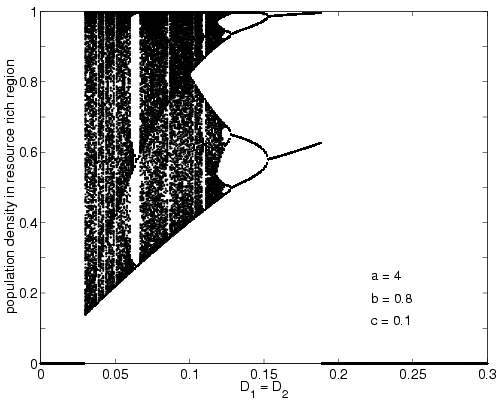
Habitat selection:
More members of a species will prefer staying in the resource rich region (source) than migrating to a resource poor region (sink). Migration will occur only when population pressure has exceeded a threshold.
We model this by switching on the coupling only when the population size difference between the two habitats exceed a finite threshold.
No qualitative change in the results; slight changes in the alteration produced by coupling in the low x region of the resultant map.

Generality of the results:
Are these results valid only for the logistic map ?
No. Similar qualitative results for the tent map.