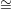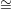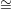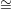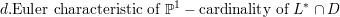A smooth projective curve C over a field k is by definition a smooth irreducible projective variety of dimension 1 over k. To begin with let us think of C ⊂ ℙⁿ for some unspecified n. We want to study intrinsic properties of C; properties that are independent of the specific way in which it is expressed as closed sub-variety of ℙⁿ. The fundamental invariant associated with C is its genus g. The genus can be defined in many different ways.

The arithmetic genus of C is defined as follows. Consider the homogeneous co-ordinate ring R of C; recall that this is the quotient of the polynomial ring k[X₀,…,X_n] (which is the homogeneous co-ordinate ring of ℙⁿ) by the (homogeneous) ideal generated by the equations defining C. The statement that C is of dimension 1 is equivalent to the statement that there is a linear subspace L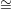ℙ^n-2 of ℙ^n-2 which does not meet C; moreover, the projection away from L gives a morphism X → ℙ¹ which has finite fibres. After a linear change of co-ordinates we may assume that L is defined by the vanishing of X₀ and X₁. The algebraic version of the above statement about C is that the ring homomorphism k[X₀,X₁] → R is injective and makes R a finitely generated graded module over k[X₀,X₁]. It follows that the dimension of R_m is a linear function of m for sufficiently large m. Writing this linear function as md + 1 - g, we note that d is the rank of R as a module over k[X₀,X₁] which is geometrically described as the number of points in the general fibre of C → ℙ¹; this is called the degree of the curve C. The number g is called the “defect” or arithmetic genus of the curve C. Of course, it needs to be shown that g is non-negative and is independent of the embedding of C in ℙⁿ.

Another notion is that of the Todd genus of C. Let (ℙⁿ)^* be the dual projective space of ℙⁿ which we think of as the space parametrising hyperplanes (linear ℙ^n-1’s) in ℙⁿ. Recall that this is expressed by writing the correspondence I in ℙⁿ × (ℙⁿ)^* which is defined by the equation ∑ _iX_iY _i = 0 if X_i’ and Y _i’s are homogeneous co-ordinates in ℙⁿ and (ℙⁿ)^* respectively. Now, consider the dual variety D_C in (ℙⁿ)^*, which is the locus of all hyperplanes that are tangent to C. More precisely, we can describe it as follows. For each point p in C, we have a line T_p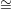ℙ¹ which is tangent to C at p. Consider the sub-variety J of I which is the locus of all pairs (p,H) where p lies on C and H is a hyperplane in ℙⁿ (and hence a point of (ℙⁿ)^*) that contains T_p. Then D_C is the image of J in (ℙⁿ)^*. If k is a field of characteristic zero or if we replace the given embedding of C by a Veronese twist, then J → D is an isomorphism over an open subset of D_C. It follows that D_C is of dimension n - 1 and is thus defined by a single homogeneous polynomial F in the Y _i’s. We write the degree of this polynomial as 2d + 2g - 2, where d is the degree of C; equivalently the Todd genus of C is defined as (deg(F)∕2) + 1 - d. Of course, one needs to show that this is a non-negative integer and independent of the embedding of C in ℙⁿ.

Assume that k is a field of characteristic 0. The coefficients of a given system of equations defining C generate a sub-field E of k which is a finitely generated extension of ℚ, the field of rational numbers. Any such field can be embedded in ℂ, the field of complex numbers. Choose such an embedding. With all these choices, we can now take the “same” curve over the field of complex numbers. As seen in other lectures, this gives a compact oriented topological manifold of (real) dimension 2. Such a manifold can be visualised as a sphere with g handles for a certain non-negative integer g which is called the topological genus. As before, one must show that this definition is independent of the various choices made above.

How does one extend the above definition to fields of non-zero characteristic. We notice that the abelianised fundamental group of C is the free abelian group on 2g generators. This motivates the following definition. Let l be a prime number different from the characteristic of k; we also assume that k is separably closed. Consider the isomorphism classes (over C) of finite smooth morphisms C′→ C which are Galois and of degree l. The number of such classes is N = (l^2g - 1)∕(l - 1). In other words the genus of C is the half of the number of “digits” required to express N in base l. As before, we need to show that this number is independent of the l chosen.

Now one way to prove that the various definitions of genus are independent of the choices made is to show that they are equal! For example, it is clear the the topological definitions do not depend on the embedding in ℙⁿ and give non-negative integers. On the other hand it is clear that the algebraic definitions are independent of any of the choices made in the topological definitions.

Let N_d denote the rational normal curve of degree d in ℙ^d; it is the image of the d-tuple Veronese embedding of ℙ¹ in ℙ^d. Let d be much larger than g and let us choose g disjoint pairs (P_i,Q_i) of distinct points in N_d. Let L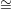ℙ^2g-1 be the linear span of these 2g points and A_i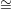ℙ¹ be the line that joins P_i with Q_i. Let M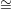ℙ^g-1 be a linear space in L that meets each A_i at a point R_i that is distinct from P_i and Q_i. Let C₀ be the image of N_d in ℙ^d-g under projection away from M. We note that (by the non-vanishing of the Vandermonde determinant), L meets N_d precisely (and transversely) in the points P_i’s and Q_i’s. So C₀ is obtained by identifying (or pinching together) the pairs of points on N_d.

Let us put this more algebraically. The homogeneous co-ordinate ring R of N_d is the sub-ring of k[U,V ] generated by all monomials UⁱV ^d-i of degree d; the assignment T_iUⁱV ^d-i gives a surjective homomorphism k[T₀,…,T_d] → R. The line A_i corresponds to a rank-2 quotient W_i of the linear space spanned by the T_i (the kernel of this map is the ideal defining A_i). The points P_i, Q_i and R_i correspond to distinct rank-1 quotients of W_i. It follows that we can think of R_i as the graph of an isomorphism between the quotients corresponding to P_i and Q_i. In other words, we obtain graded homomorphisms P_i : R → k[P] and Q_i : R → k[Q] and R_i is a graded isomorphism between k[P] and k[Q]. Then the co-ordinate ring S of C₀ is the sub-ring of R consisting of those elements F such that for each i the image of F under P_i and Q_i are identified under the isomorphism given by R_i. It is thus clear that the rank of the graded piece S_m is precisely g less than the rank of the graded piece R_m. Since the latter has rank precisely md + 1, it follows that C₀ is a (singular) curve with arithmetic genus g.

Now, suppose we could show that as we “deform” C₀ in ℙ^d-g, the singularities of C₀ will disappear. Moreover, suppose we could show that such deformations “reach” all curves of arithmetic genus g in ℙ^d-g. These two statements can be proved by the use of Hilbert schemes (which were introduced in the previous lecture). Assuming these results let us see why the topological genus of the curves so obtained is also g.

How does one deform away the singularity of C₀? To understand this let us see how one can obtain C₀ from a smooth oriented compact manifold of (real) dimension 2. As noted above, such a manifold can be visualised as a sphere with g handles. Let us choose a loop around each handle and collapse this loop to a point. We see that we obtain a surface of the same topological (homeomorphism) type as C₀.

To understand the relation between the Todd genus and the topological genus, we note that a general line L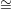^*ℙ¹ in (ℙⁿ)^* corresponds to a linear subspace L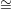ℙ^n-2 in ℙⁿ; each point of L corresponds to a hyperplane in ℙⁿ that contains L. Now, as we mentioned above, a general such L does not meet C and projection away from L can be identified as a morphism f : X → L^*; the fibre over a point p in L^* being the intersection of the corresponding hyperplane with C. As we saw earlier, f has degree d. Now, the intersection of L^* with the dual variety D_C corresponds precisely to those hyperplanes that meet C tangentially. For a general choice of L the intersection of D_C and L is transversal; this means that the hyperplane corresponding to this intersection has exactly d - 1 points with tangency at one of these points. (This is called a Lefschetz pencil of hyperplanes for the curve C). Let us choose a triangulation of L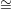^*ℙ¹ such that the points of L^*∩ D are vertices in this triangulation. We then see that the Euler characteristic of C is

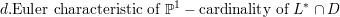
For a compact oriented manifold of (real) dimension 2, the Euler characteristic is 2 - 2g, thus we see that the Todd genus and topological genus are the same.

The assertion that the Todd genus is the same as the arithmetic genus can be seen as the principal assertion of the Riemann-Roch theorem for curves (which also asserts a bit more!). In particular, Hirzebruch and Grothendieck were able to generalise much of the above through their extension of the Riemann-Roch theorem. Numerous simpler proofs of this theorem have been given since Hirzebruch and Grothendieck first published their proofs; notably one by V. K. Patodi using differential geometry and one more recently by M. V. Nori which is purely algebraic.

Let X ⊂ ℙⁿ be a smooth projective variety and R be its homogeneous co-ordinate ring. Since this is a finitely generated graded ring over k, the rank of R_m is a polynomial function of m for all sufficiently large m. To express the coefficients of this polynomial in terms of certain intersection numbers like the degree of X, the degree of the dual variety of X and so on is the consequence of these generalisations of the Riemann-Roch theorem for curves. At the same time, such intersection numbers should be computable using topological properties of X.

As an exercise compute the different genuses for a plane curve.